WATERMAN 5

waterman 1-8 sphere clusters;
radial sweeps in fcc with increments of the square roots of sucessive integers...spheres are all diameter 1.

Other Waterman clusters and polyhedron pages... 1 2 3 4 5 6 7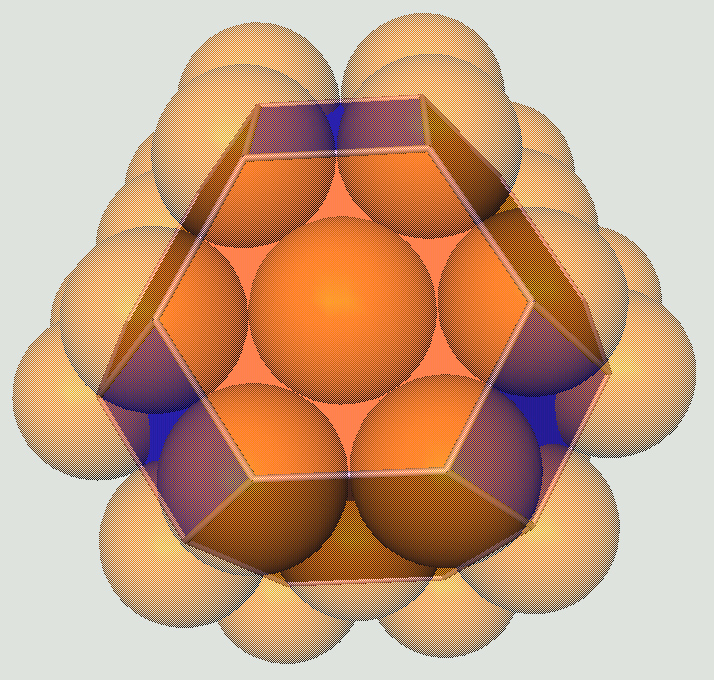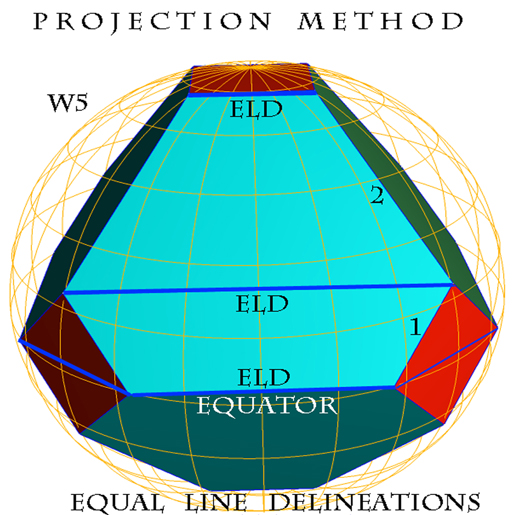



This one above, named W5, was selected as it was best to miminize land breaks, called sinuses.



The spheres act to determine the polyhedra shape. The centers are connected to make a convex hull.


The blue lines, are drawn to go all the way around the polyhedra.
That total length is then divided into 72 equal portions.

Longitudes are then drawn from the pole to the square edges.
These then continue down to the longest blue lines; itself also divided into its own equal 72 lengths.
To complete the longitudes, we continue to drawn lines to the likewise equally divided equator.

Last, is to divided the total lengths of longtudinal sections from pole to pole into 36 equal lengths.
The final projection grid for the W5, having longitude and latitude lines at 5 degree intervals...